Combinatorics and the Mandelbrot Set
Faculty advisor: Sarah Koch
Grad student mentor: Jasmine Powell
Undergraduate researchers: Mitch Boyce, Yi Lu, Jonathan Thomas
Given a quadratic polynomial of the form f_c(z)=z^2+c with c a complex number, we can iterate the function and look at the behavior that arises. For example, if we plot all values of c in the complex plane so that the orbit of 0 under iteration of f_c remains bounded, we get the famous Mandelbrot set. In this project, we will look at specific values of c that lie in the Mandelbrot set, and compute algebraic data associated to the polynomials f_c, using the combinatorics of the Mandelbrot set as a guide for looking for patterns in this data.
Here is the final poster from this project. Here is one image produced in the course of the project: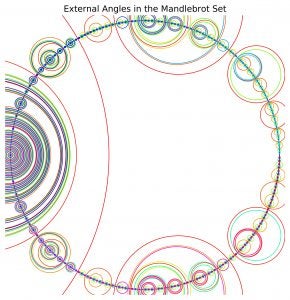
This is a visualization of the external angles in the Mandelbrot Set. The Mandelbrot set has parameter rays running toward it from infinity, and each ray has an associated angle it makes with the real axis. This picture shows the angles of pairs of rays that meet at root points of the Mandelbrot set.
Visualizing the Schwarzian Derivative
Faculty advisor: Dylan Thurston
Grad student mentors: Patrick Haggerty, Didac Martinez-Granado, Maxime Scott
Undergraduate Researchers: Noah Luntzlara, Piriyakorn Piriyatamwong, Mengxi Wang, Sharon Ye
Given a holomorphic function f on the complex plane, the Schwarzian derivative S(z) measures how much f deviates from a Mobius transformation. This deviation can be captured by 3-dimensional pictures that show how an extension of the map f distorts hyperbolic space. In this project we will look at specific holomorphic maps and study their Schwarzian derivatives visually, to see what kind of geometric information we can extract.
Here is the final poster from this project. Below is one of the images produced by this team:
Pictured above is the foliation by annuli generated by the osculating circles of the logarithmic spiral, which arose in this project while discussing the notion of curvature and trying to relate it to the Schwarzian derivative. This picture shows that when the curve has a monotone increasing curvature, the osculating circles do not intersect.
Geodesics on Hyperbolic Surfaces
Faculty advisors: Caleb Ashley and Patrick Boland
Grad student mentor: Feng Zhu
Undergraduate researchers: Karl Kirchner, Jimin Oh, Rebecca Su
In this project, we investigate a bit of Riemann surface theory with intersections with hyperbolic geometry, dynamics and number theory. (See C. Series “The Geometry of Markoff Numbers,” and work cited there for more background.) In particular, we explore properties of geodesics on the modular torus, H^2/Γ′ and the thrice punctured sphere, H^2/Γ(2). We seek answers (with emphasis on visual demonstrations) to quan- titative and qualitative questions such as; How many simple closed geodesics are there? How does the number of self intersections increase with length, or the amount of time spent in cusp?
Click here to see the final poster for this team’s project.


