Research
Overview
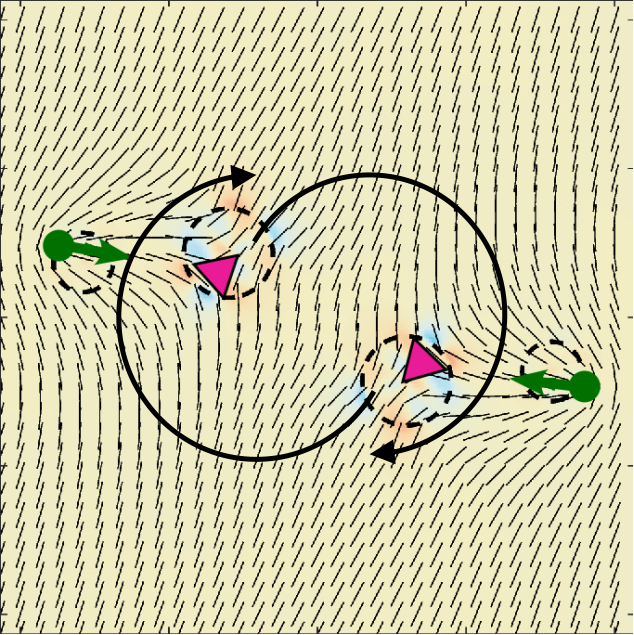
We work on a wide range of problems at the interface of physics, mathematics, and biology. Our primary interest is in asking how collective properties emerge and become functional in inert and living materials. The focus is thus on obtaining a qualitative, quantitative, and predictive understanding of how complex patterns form, what their nonlinear dynamics is, and how they can be controlled in artificial (engineered) or living (biological) systems. To do this we leverage a variety of tools from continuum mechanics (e.g., elasticity, hydrodynamics), condensed matter (e.g., scaling, phase transitions), and mathematics (e.g., geometry, topology, optimization) to develop minimal theoretical models, perform numerical computations, and build simple macroscopic experiments to explain and control emergent collective phenomena. We have been fortunate to collaborate closely with researchers from across the world on a variety of problems, ranging from the dynamics of motile colloids and bacterial suspensions to the mechanics of torn paper to the morphogenesis of visceral organs.
Click on the tabs below to learn more in detail about the various research directions.